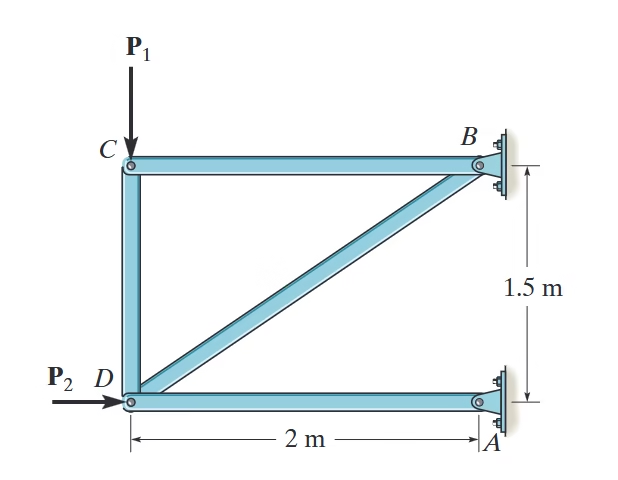
$6–1.$ Determine the force in each member of the truss and state if the members are in tension or compression. Set ${P_1} = 20 kN$, ${P_2} = 10 kN$
$ \text{Step by Step Solution} $
$ \text{Step 1: Given Data} $
We are tasked with determining the forces in each member of the truss and identifying whether these forces are in tension (T) or compression (C).
\begin{array}{c}
\text{Forces:} \\
P_1 = 20\,\text{kN} \\
P_2 = 10\,\text{kN}
\end{array}
$ \text{Step 2: Joint C Analysis} $
The free-body diagram of joint C is shown below:

\begin{array}{c}
\text{Equilibrium in x-direction:} \\
\sum F_x = 0 \\
-F_{BC} = 0 \\
\hline
F_{BC} = 0\,\text{kN}
\end{array}
\begin{array}{c}
\text{Equilibrium in y-direction:} \\
\sum F_y = 0 \\
F_{CD} – P_1 = 0 \\
F_{CD} = P_1 = 20\,\text{kN (T)}
\end{array}
$ \text{Step 3: Joint D Analysis} $
The free-body diagram of joint D is shown below:

\begin{array}{c}
\text{Equilibrium in y-direction:} \\
\sum F_y = 0 \\
-F_{CD} – F_{BD} \times \frac{3}{5} = 0 \\
F_{BD} = -F_{CD} \times \frac{5}{3} \\
= -20 \times \frac{5}{3} \\
= -33.33\,\text{kN} \\
= 33.33\,\text{kN (C)}
\end{array}
\begin{array}{c}
\text{Equilibrium in x-direction:} \\
\sum F_x = 0 \\
P_2 – F_{AD} – F_{BD} \times \frac{4}{5} = 0 \\
F_{AD} = P_2 – F_{BD} \times \frac{4}{5} \\
= 10 – (-33.33) \times \frac{4}{5} \\
= 36.7\,\text{kN (T)}
\end{array}
$ \text{Conclusion} $
The forces in each member are:
\begin{array}{ll}
\text{Member BC:} & 0\,\text{kN} \\
\text{Member CD:} & 20\,\text{kN in tension (T)} \\
\text{Member BD:} & 33.33\,\text{kN in compression (C)} \\
\text{Member AD:} & 36.7\,\text{kN in tension (T)}
\end{array}

Leave a reply